SEMILINEAR PARTIAL FUNCTIONAL DIFFERENTIAL EQUATIONS WITH THE NONLINEAR OPERATOR IS LIPSHITZ
Abstract
On a Banach space X, we consider the semilinear partial functional differential equations of the form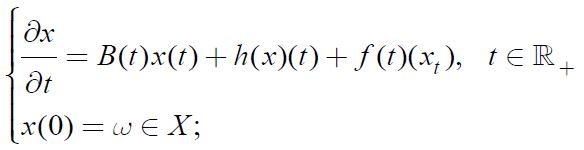
where the mapping t → B(t) is a possibly unbounded operator such that the family (B(t))t≥0 generates an evolution family (U(t, s))t≥s≥0 have an exponential dichotomy; the operator h : BC(R+ , X ) → BC(R+ , X ) is Lipshitz; f (t)∈L(M, X ) ; xt is the history function defined by xt (s) := x(t + s) for s ∈[-θ , 0] . By using the Lipschitz of the nonlinear operator and the inequalities of evolution family in Banach spaces, we prove the equivalence of the two forms solutions to equation (1) as a mild solution and a Lyapunov-perron solution.
References
J.L. Massera, “The existence of periodic solutions of systems of differential equations”. Duke Math. J., 1950, Vol. 17, pp. 457-475.
J. K. Hale, O. Lopes,”Fixed point theorems and dissipative processes”. J. Diff. Equ., 1966, Vol. 13, pp.391-402.
R. Benkhalti, H. Bouzahir, K. Ezzinbi , “Existence of a periodic solution for some partial functional differential equations with infinite delay”, J. Math. Anal. Appl., 2001, Vol. 256, pp. 257-280.
R. Benkhalti, A. Elazzouzi, K. Ezzinbi, “Periodic solutions for some partial neutral functional differential equations”. Elec. J. Diff. Equ., 2006, Vol. 56, pp. 1-14.
J.J. Massera, J.J. Sch¨affer, Linear Differential Equations and Function Spaces, 1966, Academic Press, New York.
A. Pazy, Semigroup of Linear Operators and Application to Partial Differential Equations, 1983, Springer, Berlin.
R. Nagel, G. Nickel, “Well-posedness for non-autonomous abtract Cauchy problems”, Prog. Nonl. Diff. Eq. Appl., 2002, Vol. 50, pp. 279-293.

